A new theory regarding how the brain first learns basic math could alter approaches to identifying and teaching students with math learning disabilities. Published in the Behavioral and Brain Sciences journal, Ben-Gurion University of the Negev (BGU) researchers offer a better understanding of how, when and why people grasp every day math skills.
The most widely accepted theory today suggests people are born with a “sense of numbers,” an innate ability to recognize different quantities, like the number of items in a shopping cart, and that this ability improves with age. Early math curricula and tools for diagnosing math-specific learning disabilities such as dyscalculia, a brain disorder that makes it hard to make sense of numbers and math concepts, have been based on that consensus.
Ph.D. students Naama Katzin and Maayan Harel and Prof. Avishai Henik, all from the BGU Department of Psychology and the Zlotowski Center for Neuroscience, collaborated with Dr. Tali Leibovich from the Numerical Cognition Laboratory at the Department of Psychology & Brain and Mind Institute, University of Western Ontario. Dr. Leibovich was formerly a Ph.D. researcher at BGU’s Department of Brain and Cognitive Sciences and the Zlotowski Center.
“If we are able to understand how the brain learns math, and how it understands numbers and more complex math concepts that shape the world we live in, we will be able to teach math in a more intuitive and enjoyable way,” says Dr. Leibovich. “This study is the first step in achieving this goal.”
The study challenges the prevalent “sense of numbers” theory. Other theories suggest that a “sense of magnitude” that enables people to discriminate between different “continuous magnitudes,” such as the density of two groups of apples or total surface area of two pizza trays, is even more basic and automatic than a sense of numbers.
The researchers argue that understanding the relationship between size and number is critical for the development of higher math abilities. By combining number and size (e.g., area, density and perimeter), we can make faster and more efficient decisions.
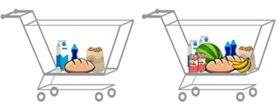
Take for example the dilemma over choosing the quickest checkout line at the grocery store. While most people intuitively get behind someone with a less filled-looking cart, a fuller-looking cart with fewer, larger items may actually be quicker. The way we make these kinds of decisions reveals that people use the natural correlation between number and continuous magnitudes to compare magnitudes.
The researchers also urge colleagues to consider the roles other factors, such as language and cognitive control, play in acquiring numerical concepts. While the theoretical models presented in this review may raise more questions than answers, the researchers hope their hypothesis will reveal new ways of identifying dyscalculia, which can currently only be diagnosed in school-aged children. By this stage, children with the disorder are already lagging behind their peers.
“This new approach will allow us to develop diagnostic tools that do not require any formal math knowledge, thus allowing diagnosis and treatment of dyscalculia before school age,” says Dr. Leibovich.